The population of any region is characterised with respect to its growth rate. Exponential and Logistic growth models are two typical but important growth models that can be used to track the population’s growth rate.
Exponential growth is characterised by the rapid expansion of the population that is unaffected by any upper limit. Contrarily, logistic growth refers to a sustainable growth rate that has an upper limit of growth.
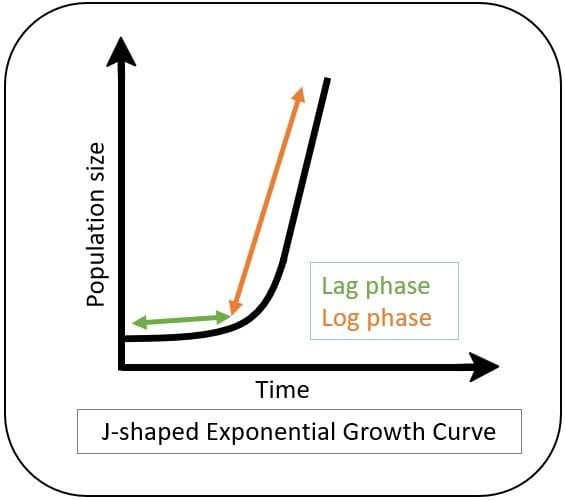
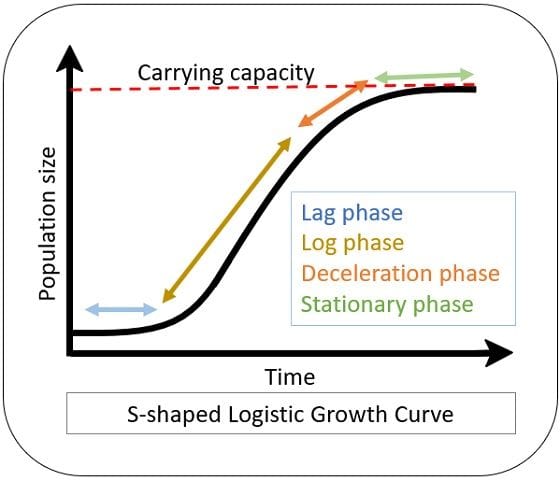
The exponential growth model depicts an indefinite growth curve in the form of a J-shaped curve. This happens when there are endless resources available like lots of food, water and territory to occupy. The logistic growth model, on the other hand, displays an S-shaped sigmoid curve. This model is particularly practical since it occurs when resources are limited, as they are on Earth.
Since the exponential growth assumes steady population amplification, it may result in a population explosion. Logistic growth is risk-free from this as it maintains a logical equilibrium between the birth and death rates.
With the use of a comparison chart, graphs, and examples, we will examine the actual differences between these two growth models in the following content.
Content: Exponential Vs Logistic Growth
Comparison Chart
| Basis of Comparison | Exponential Growth | Logistic Growth |
|---|---|---|
| Meaning | Exponential growth portrays the constant rise in the population number over a certain period of time. | Logistic growth depicts the growth where the population rises initially but then gets saturated at a certain point. |
| Growth model | J-shaped growth model. | S-shaped (sigmoid) growth model. |
| Birth rate | Birth rate is directly proportional to the time and size of the population. | Birth rate increases at the beginning but then gradually starts decreasing with an increase in population. |
| Occurrence | It takes place in the abundance of resources like food, land etc. | It occurs in the limited supply of resources. |
| Upper Limit | There is no upper limit and thus, the population keeps on growing. | There is an upper limit known as carrying capacity, which restricts the overgrowth. |
| Growth Rate | Growth rate of the population may pass the carrying capacity limit. | Growth cannot exceed beyond the carrying capacity of that ecosystem. |
| Population status | Due to the sudden and massive mortalities, the population often crashes. | Crash in the population is rarely observed. |
| Population explosion | Leads to the population explosion due to high amplification number. | Explosion is seldom seen due to the sustained and constant growth rate. |
| Stationary phase | Rare or absent | Present |
| No. of phases | All four phases are present: • Lag • Log • Deceleration • Stationary | Only two phases are dominant: • Lag • Log |
| Applicable to | Population has no limiting agents with plenty of resources. | Population with carrying capacity. |
What is Exponential Growth?
The exponential growth model depicts the growth of the population size at a constant rate over a set length of time. In this type of growth, the birth rate is directly proportional to the size of the population as well as to the time. After plotting a graph between population size and time period, the J-shaped curve is obtained due to the uninterrupted amplification of population size.
Mathematical equation for Exponential Growth
Characteristics of the Exponential Growth
- Here, the population has access to an infinite amount of resources. For example, where there is an infinite amount of food to eat, ample sunlight to absorb, an inexhaustible supply of water, and an endless area to cover.
- Here, the population is not influenced by the presence of competitors and predators.
- Such a growth model lacks a predetermined upper limit to prevent overpopulation.
- Since every individual’s growth rate remains constant regardless of population size, organisms are thereby added in every generation, leading the population to increase faster.
- At the initial growth stage, the doubling rate is quite low due to the lesser number of reproducing organisms. But as the number rises, the population experiences exponential growth.
Examples of the Exponential Growth
This kind of growth is mainly observed in the areas that are newly constructed by the species or in areas that have been entirely devastated due to a natural disaster.
- One of the suitable illustrations of exponential growth is a bacterial or fungal culture growing in the laboratory. Here, the microbes proliferate by feeding on the provided media. Their population continues to grow until the nutrients available to them are depleted.
- Another example might be a forest that is regrowing after being completely destroyed by a forest fire. The new species that emerge in such an environment will have unrestricted access to sunlight, food, and water, as well as the entire landmass to populate. These species will spread until they have completely covered the area and depleted the resources.
Point to be noted: It’s worth noting that if the population is allowed to increase exponentially without restraint, the entire globe will be engulfed in a matter of months. Nature, on the other hand, cannot simply enable anything to flourish unchecked indefinitely. And ultimately, there is no such thing as infinite resource availability. Some items will eventually get exhausted as a result of their excessive use. As a result, the exponential growth model is hard to find or even found; won’t last long.
What is a Logistic Growth?
Logistic growth depicts growth where the population increases slowly and then saturate at a certain limit. Here the growth rate decreases with an increase in population in a specific time period. The logistic growth is influenced by several limiting agents like the carrying capacity of an ecosystem, limited availability of resources, presence of predators and competitors etc. Due to these factors, S-shaped graph is achieved after plotting population growth against time.
Logistic growth is a type of growth in which the population grows slowly and finally reaches a saturation point. In this case, the growth rate slows as the population grows over time.
Several limiting agents affect logistic growth, including an ecosystem’s carrying capacity, restricted resource availability, predators, competitors, and so on. After graphing population growth against time, an S-shaped graph is produced as a result of these factors.
Mathematical equation for Logistic Growth
Characteristic of Logistic Growth
- This type of growth model is obtained in the limited supply of resources like a finite quantity of food, water as well as limited space as shelter.
- Here the population size is affected due to the competitors and predators.
- Logistic growth has an upper limit known as carrying capacity. The logistically growing population cannot pass beyond this upper limit.
- In such growth, the population size rises gradually, and as soon as will each near the carrying capacity, it will start to slow down.
- Eventually, the growth curve will plateau or flatten, making S-shaped curve.
Examples of Logistic Growth
The African elephants were on the edge of extinction in 1900, and the African government created regulations to prohibit poaching at the time. After a few years, their population reached its peak due to no competition and plenty of resources. However, as the population grew, competition for resources, mating partners, and territories grew as well. Due to this, their population began to dwindle once more. Nature maintains this natural increase and decrease in population numbers. Thus, this is a good illustration of logistic growth.
Factors affecting the population growth
Limiting factors are those that have a direct or indirect impact on population increase. It might be a biotic or abiotic element that controls and limits the birth rate and distribution of organisms in a certain area. They are the ones who decide how big a population can get. The following are some of the primary limiting factors:
- Resource availability: All organisms require food, water, and sunlight to survive. Similarly, they require a shelter where they may thrive. All of these are referred to as resources. Any population will not be able to grow appropriately if any of these are insufficient. In such cases, individuals will perish from malnutrition, stress, or desiccation, leading to extinction.
- Carrying capacity(K): It can be defined as the maximum number of organisms that the environment can endorse for survival. The growth and size of the population constantly fluctuate as both the limiting factors and nature are bound to sustain the environmental balance. This fluctuation gives rise to the concept of carrying capacity.
- Predation: The predators too limit the population size. They consume the organisms as food for the sake of survival. This eventually keeps their total under the acceptable range.
- Competition: competitors are individuals who will battle with one another for the same resources in order to survive. Because resources are limited, competition for them will slow the rate of growth.
For example, in the forest, trees constantly growing one on top of the other will not cut one another but will eventually cut each other off from sunshine.
Key Differences between Exponential and Logistic Growth
- Exponential growth is rarely possible since it needs idealistic conditions like an unlimited supply of nutrients, water, sunlight and endless space to capture. Such situations tend not to appear in the practical world. But in some of the exceptional cases, this population model is observed.
In contrast, the logistic growth model is quite practical and may be found around you. This is a typical growth model that is impacted by biotic and abiotic agents, has a finite amount of food, water, and light, and has a finite amount of area to inhabit. - In the exponential growth model, the population size continues to grow forever without any boundation, resulting in a J-shaped curve. This growth curve plateaus or flattens in logistic growth and becomes sigmoid, resulting in an S-shaped curve.
- The population has unrestricted access to resources and can expand to its full biotic capacity while growing exponentially. This is feasible since they have no upper limit and can quickly exceed the carrying capacity index due to a lack of competition and an abundance of resources.
- Populations with exponential growth have the potential to explode at some point. This explosion might cause a sudden collapse of the population. On the other side, the logistic population is rare to be seen under such situations due to their long-term stability.
- In the exponential growth model, the birth rate is directly proportional to the time and size of the population. As a result, the number of people continues to rise as time passes. But during logistic growth, the pace of population growth increases at first but then slows when resources become scarce.
Summary
The population of a particular region is characterised on the basis of its growth rate. The growth can be observed with the help of two growth models- exponential and logistic.
In the case of unlimited resources and no competition, exponential growth leads to perpetual population expansion with no upper limit. The logistic growth rate is organically maintained and occurs in a finite number of resources. It has the highest limit of growth called as carrying capacity.
Both models are used to research population growth. However, because nature doesn’t allow limitless growth of anything eternally, the logistic growth model is far more practical than the exponential.


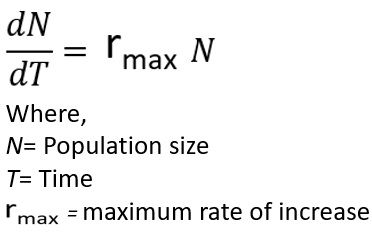
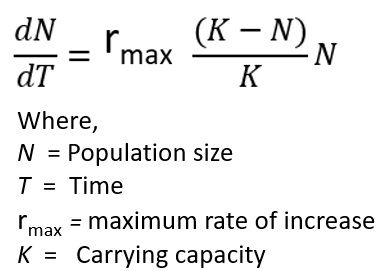


Leave a Reply